Confidence Interval for a Population Proportion | Standard Normal Curve| Leaving Cert Maths |

Task:
Find the 95% confidence interval for the proportion of new cars on this road.
Give your answer correct to 4 significant figures.

Given information:
A motoring magazine collected data on cars on a particular stretch of road.
Certain details on 800 cars were recorded.
The ages of the 800 cars were recorded. 174 of them were new (less than 1 year old).

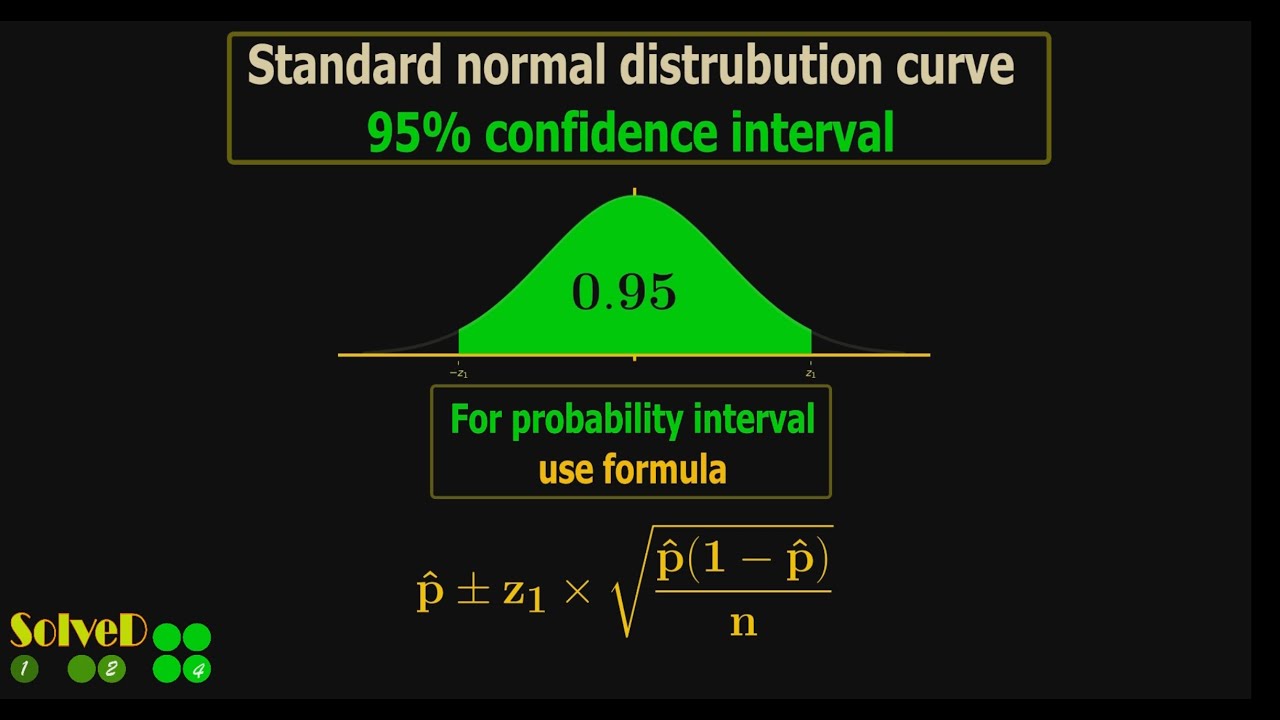
95% confidence interval will give the probability range (interval) that a randomly selected sample, out of all 800 cars, is going to be a new car.

The formula for a confidence interval (CI) for a population proportion is shown.

p^ is the sample proportion, n is the total data size, and z1 is the
z-value from the standard normal distribution relative (corresponding) to the desired (required)
confidence level.

Step1: Find the sample proportion,p^.
Step2: Find the value of z1.
Step3: Find margin of error = z1 times square root [p^(1-p^)]
***************************************
Contents:
(1) Problem description
(2) Method
(3) Solution
(4) Key points summary
***************************************
Leaving Cert | HL| Exam | 2019 B | Q8(a) (i)|
***************************************
#LeavingCertMaths
#SolveD124 | SolveD124

confidence interval,margin of error Standard Normal Distribution Curve,Standard Normal Curve,z score,z value,comparison using z values,probability,statistics,Junior and Leaving Certificate Maths,Leaving cert maths,Leaving cert,maths,junior cert,GCSE,GCSE maths,leaving cert,irish leaving cert,leaving cert notes,Leaving Cert Maths,igcse maths,maths class 10,leaving certificate,class 12 maths,